Welcome, students, to another comprehensive study guide designed to support your journey toward success. This time, we turn our focus to the module “Applied Algebra WGU C957 ,” breaking it down into easily digestible segments. Our aim, as always, is to provide you with all the necessary information to comprehend the module and navigate through it effectively, ensuring your success along the way.
Every piece of information presented in this article has been meticulously curated through thorough research and the invaluable insights of former students. Our goal is to equip you with the most accurate and valuable tips and guidance to ease your experience with this WGU C957 course. By following our advice, passing this module will become a straightforward task.
Throughout this article, we will delve into:
With this comprehensive guide at your disposal, you’re well-equipped to tackle the challenges of the “Applied Algebra WGU C957” module with confidence and achieve your academic goals. Let’s dive in and pave the way for your success!
...
What to expect from Applied Algebra WGU C957 module 📖
The module “Applied Algebra WGU C957” is an essential component of your academic journey, offering a deep dive into the practical application of algebraic principles in real-world scenarios. Throughout this course, you will embark on a comprehensive exploration of how algebraic functions, graphs, and models can be effectively utilized to analyze and interpret data, providing you with invaluable skills applicable across various disciplines and industries.
Central to the course curriculum is the development of proficiency in working with functions and understanding their underlying algebraic properties. You’ll begin by exploring the diverse applications of functions in real-life contexts, gaining insights into how they can be harnessed to address practical challenges encountered in fields ranging from economics to engineering.
The curriculum encompasses the study of several families of functions, and the content is broken down into below topics;
- Topic 1: Introduction
- Topic 2: Function Interpretation
- Topic 3: Meanings of Functions
- Topic 4: Linear Functions
- Topic 5: Polynomial Functions
- Topic 6: Exponential Functions
- Topic 7: Logistic Functions
- Topic 8: Graphical Depictions
- Topic 9: Validity of Models
Moreover, you’ll learn to leverage these mathematical tools to solve complex problems relevant to your academic pursuits and everyday life. Whether it’s optimizing resource allocation in business or predicting population growth in demographics, the skills acquired in this module will empower you to make informed decisions and navigate real-world challenges with confidence.
It’s worth noting that prior knowledge in Applied Probability and Statistics or its equivalent is recommended before enrolling in Applied Algebra, ensuring you have a solid foundation to build upon. Additionally, for your convenience during assessments, it is advisable to have a calculator handy for any necessary calculations.
By engaging with the content and applying yourself diligently, you’ll emerge from this module not only with enhanced mathematical proficiency but also with a newfound appreciation for the practical relevance of algebra in shaping our understanding of the world around us.
...
Study Guide for Preparing for the OA 📝
Now that you are aware of what to expect from this module, let us proceed to discuss how to tackle the course content and ace the OA in the most efficient way. With our study guide, we have eliminated the need for you to comb through all the available study resources and worry about which advice to take and what steps you need to take next. We have done all the hard work for you, and we have figured out the best study path to achieve your goals in the shortest amount of time possible. As this module is known to be relatively easy compared to other modules in WGU, we have constructed a study guide, that will allow you to complete the course in just two weeks. So take out your notebooks and calculators, let’s get started,
Week 1 – Step 1
As we have already discussed what to expect from the module, you should have an overall understanding of the curriculum that we are about to face. So let us dive into the course content head first.
The first study material we will use for this module is the “Live Cohort Recordings by Professor Odin”, which consists of 4 videos that last about 3 hours in total. The reason we picked this resource first before the Textbook is that, the video series acts as a perfect introduction to the course content. The Instructor who is conducting these videos does a perfect job in explaining each topic and he teaches the course content with a focus on the OA, which will help you immensely in understanding which key concepts to focus on for the best outcome.
You can find the cohort videos by following the below steps;
1) Log on to the homepage of the WGU website with your student credentials
2) Navigate to the “Degree Plan” section from the Homepage
3) Click on the “Applied Algebra WGU C957” link to go to the module page
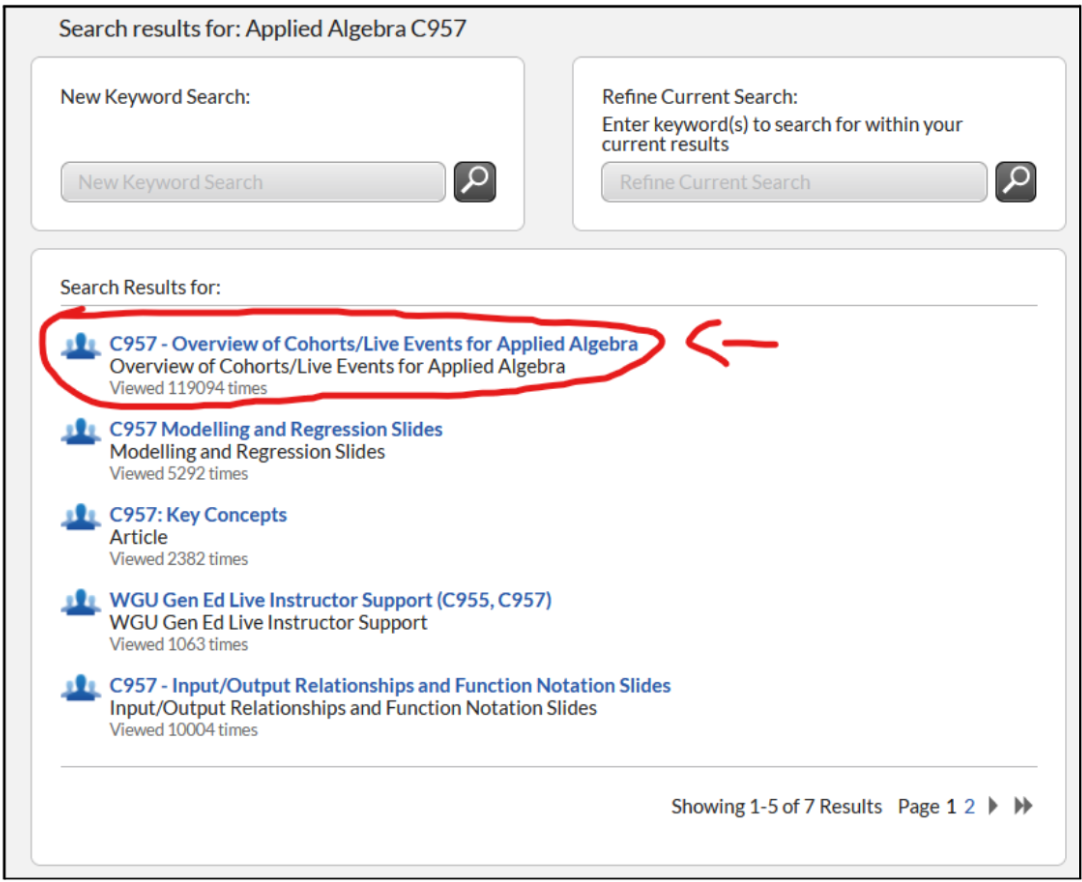
4) Go to the course search section (Refer to the image below)

5) Click on the “C957 – Overview of Cohorts/Live Events for Applied Algebra” (Refer to the image below)
When you are going through these videos, take your time to grasp the knowledge. If required re-visit each video until you are left with no doubts of the knowledge you have been given. It is advisable to take short notes during this step as well. This will help you when revising the key concepts before taking the OA. Note down the formulas and the terms and definitions that you will need to memorize on your short notes and re-read to commit them to memory.
Another important task to perform while going through the videos is to put the formulas you learn in each topic into practice. As this module deals with math equations, only memorizing the formulas won’t help you pass the OA. You should be prepared to put the formulas into practice and find the solution to a given scenario. In the video series, the professor will take you through some quizzes as well, make sure to pause the video at this point and do the calculations yourself first, before the professor takes you through the answer. Also after the end of each topic, pause the video and go to your textbook provided by WGU. There you will find extra quizzes that you can attempt at the end of each topic. Make sure you go through each of these questions before starting the next topic in the cohorts. The TextBook can be found in the Study Material section of the module page.
Week 1 – Step 2
After going through the cohort videos, you should have a clear knowledge of 80% of the course content. There are two topics still remaining for you to study which the cohorts do not discuss. To review these topics, go to the TextBook provided by WGU for module C957, and find these topics to read through them. Instead of just reading through these specific topics, we recommend you read through the entire textbook if the time allows you to do so. This will give you a chance to revise what you have learned so far as well as fill in gaps in your knowledge. As we are trying to complete the entire module in 2 weeks, this will ensure that you will be well-prepared to face the OA and achieve success in the first attempt. While going through each topic, re-try the quizzes as well to revise your memory on the equations.
Week 2 – Step 1
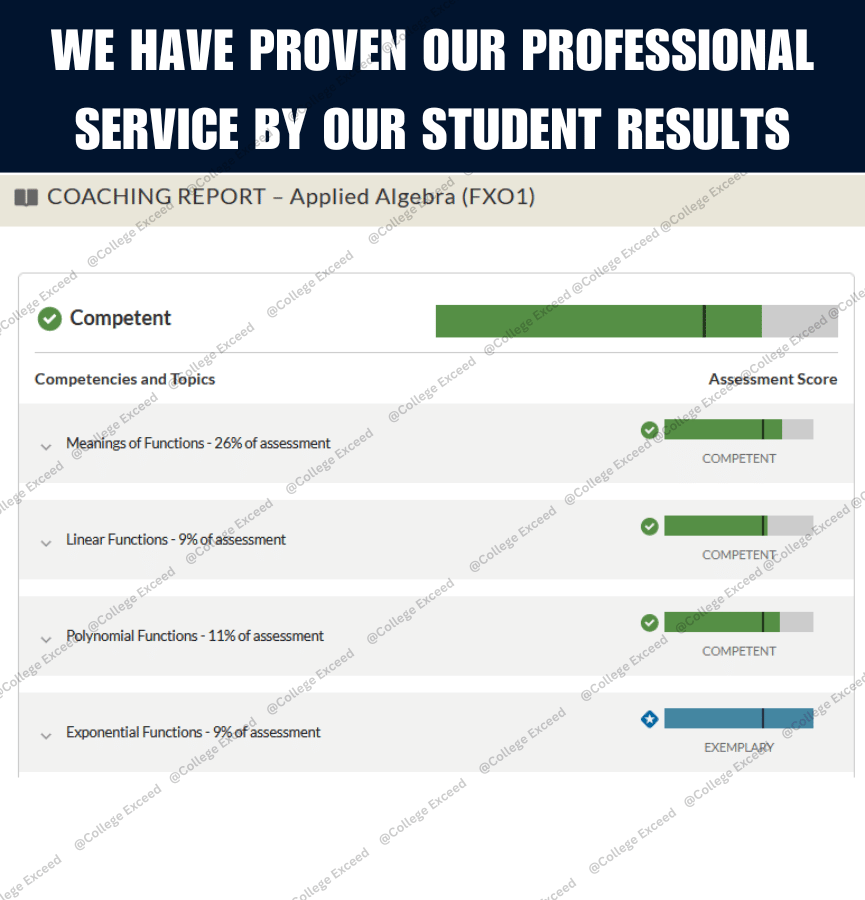
After completing both step 1 and step 2, you should have acquired the necessary knowledge to take on the assessments and put your knowledge to the test. To verify that you have gone through all the key concepts in this module, go to the “Course Search” section of the module and find the “Key Concepts” file. Download the file and go through it once to verify your knowledge. Then we advise you to go through your short notes once more to revise the topics, as we are about to attempt the Pre-A of Applied Algebra WGU C957.
Once you are confident, you should take the Pre-A and test your knowledge of the module.
To take the Pre-A, you should:
- Find a quiet and comfortable place where you can focus and avoid distractions.
- Set aside at least an hour to complete the Pre-A
- Treat the Pre-A as if it were the real OA and do your best.
- Use common sense and logic to answer the questions, not just memorization.
To review the Pre-A, you should:
- Use your coaching report to identify the competency areas and topics that you need to improve on
- Expand each competency area to see the suggested study topics and resources.
- Re-watch the videos and go through your short notes to fill the gaps in your knowledge
- Attend a live cohort (click “Explore Cohort Offerings” to enroll) and interact with the instructor and other students.
- Schedule a session with your course instructors to go through your weak points
If you have failed the Pre-A on your first attempt, re-take it and try your best once more after reviewing your weak points. Once you successfully pass the assessment, move on to the next step.
Week 2 – Step 2
We have finally arrived at the last step of the module. There is nothing else left to do but attempt the objective assessment (OA) of C957. You have already proved that you are prepared to face the final challenge by passing the Pre-A in the above step. So be confident in your abilities and schedule the OA without hesitation.
Tips for the OA
- Make sure to slow down and read the questions carefully. There are often questions that are phrased very specifically.
- Make sure you are comfortable using a calculator. This will be your main tool that you will rely on the exam.
- Memorize the order of operations. You can use the PEMDAS acronym to help you commit it to memory (Parenthesis, Exponents, Multiplication, Division, Addition, and Substraction).
- Get comfortable using Euler’s number (The “e” button on calculator) as you will see it a few times on the OA.
To take the OA, you should:
- Schedule the OA at a convenient time and date for you
- Make sure you have a stable internet connection, a webcam, and a microphone.
- Follow the proctoring instructions and rules carefully.
- Use the same strategies and skills that you used for the Pre-A
- Relax and be confident in your abilities.
Good luck!!
...
External Resources to Study📂
If you feel the provided resources above are not sufficient enough for you to understand the key concepts of this module, or you need extra study material to memorize the material, we have listed some external materials below that might assist you. Use these extra resources to aid you in your journey.
- Quizzlet #1
- Quizzlet #2
- Algebra Boot Camp (Refer to the relevant topics in the video chapters)
...
Key Topics to Focus On🎯
Functions and Types
What is a function: –
A function of mathematical relation is an association of inputs (with notation of x) and outputs (denoted by y). The input is linked to exactly one of the outputs. In short, it can be explained as a rule that gives every input value to a single assigned output value.
For example, consider the function f(x)=2x+3f. This function takes an input xx, multiplies it by 2, adds 3 to the result, and then gives us the output yy. So, if we input x=4 into the function, we get f(4)=2×4+3=11. Similarly, if we input x=−1, we get f(−1)=2×(−1)+3=1.
Functions are essential in mathematics because they help us describe and analyze relationships between quantities. They are used in various fields, including algebra, calculus, physics, engineering, and economics, to model real-world phenomena and solve problems.
Function notation: –
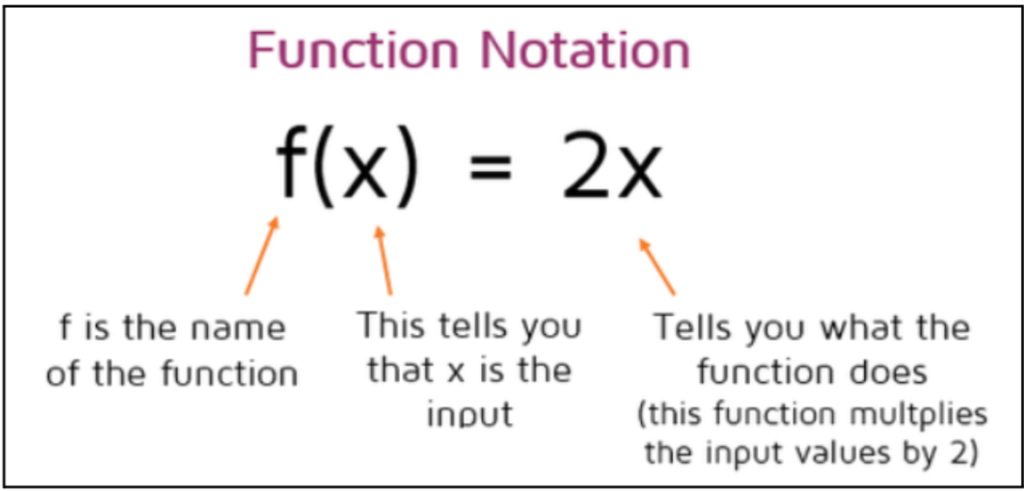
Function types: –
1. Linear Functions:
These functions represent a straight line when graphed and have the general form f(x)=mx+b, where m is the slope of the line and b is the y-intercept. Linear functions describe relationships that change at a constant rate. An example is f(x)=2x+3, where the slope is 2 and the y-intercept is 3.
2. Polynomial Functions:
Polynomial functions consist of one or more terms involving variables raised to whole number exponents. They can take various forms, such as quadratic (degree 2), cubic (degree 3), quartic (degree 4), and so on. An example of a quadratic polynomial function is f(x)=x2−4x+3.
3. Exponential Functions:
Exponential functions have the form f(x)=a.bx, where aa is a constant (the initial value or y-intercept) and bb is the base of the exponential expression. These functions model exponential growth or decay, where the rate of change is proportional to the function’s current value. An example is f(x)=2.3x, where a=2 and b=3.
4. Logistic Functions:
Logistic functions describe growth constrained by a limiting factor. They have an S-shaped curve and are commonly used to model population growth, resource utilization, or the spread of diseases. The general form of a logistic function is f(x) = L , where L is the limiting value, k is the growth rate, and 1+e−k(x−x0)
x0 is the x-value of the sigmoid’s midpoint. An example is the logistic growth model used in population dynamics.
Asymptotes
Asymptotes are imaginary lines that a graph approaches but never touches or crosses. They indicate the behavior of a function as the input approaches certain values, typically as it moves toward positive or negative infinity.
There are three types of asymptotes:
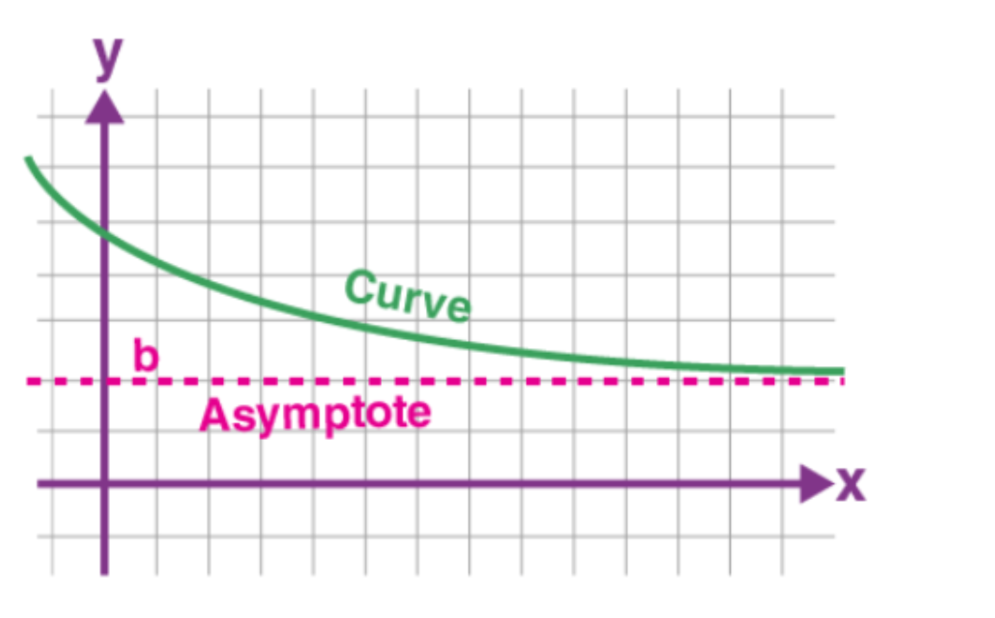
Horizontal Asymptote: A horizontal asymptote is a line parallel to the x-axis such that the curve goes to the line as the input values go to infinity or as they go to zero. The values of the function’s arguments may approach to the horizontal line, but they never hit it. In horizontal asymptotes lies the specification of the behavior of a function on the long-term. Such as the function f(x)=1x, then has a horizontal asymptote at the root of y=0.
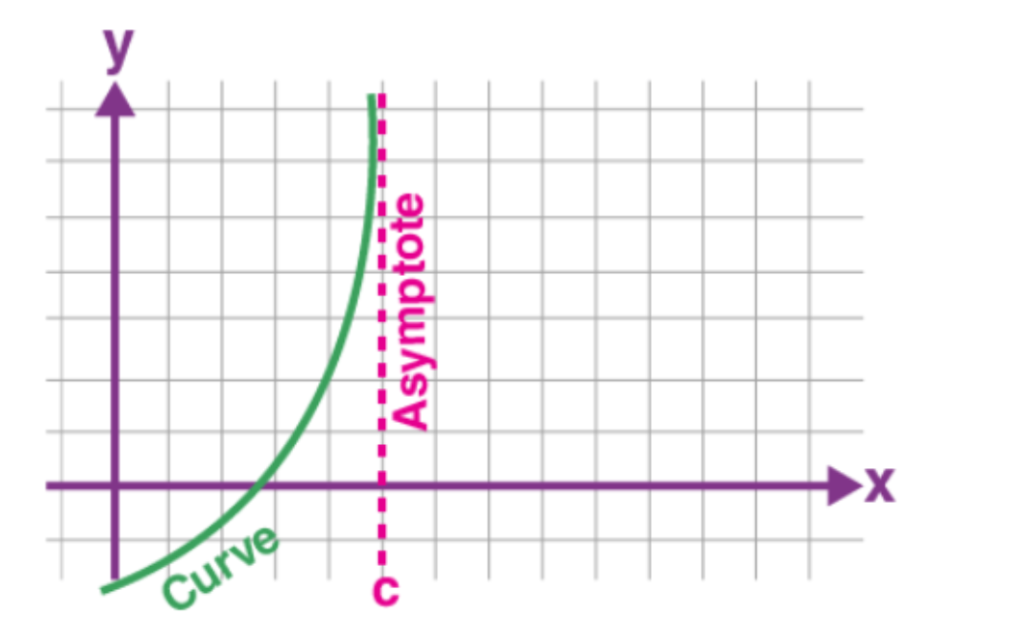
Vertical Asymptote: A vertical asymptote is a vertical line through which the graph reaches as values of the input nearly approach a certain value. The function’s value grows without limit as it nears the vertical line, but it never comes across it. Vertical asymptotes arise if the function’s denominator is zero and in that case, the function grows incomprehensible. For instance, the limit function f(x)=1x has a vertical asymptote at x=0.
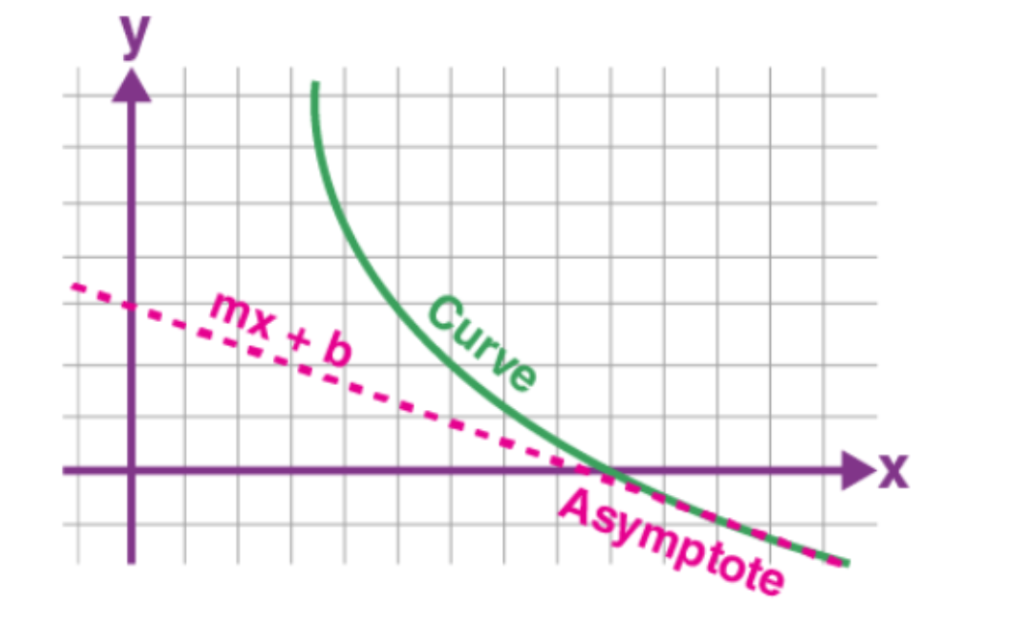
Oblique (Slant) Asymptote: A slope that is other than vertical getting data points to approach it as the input values become indefinitely large or indefinitely small. Obligatory asymptotes, unlike horizontal and vertical asymptotes, are not always encountered in all functions. These happen when the numerator of a rational function is the one with the highest degree. is greater than the numerical value of the numerator. As an illustration, the line y=x is an oblique asymptote for the function f(x)=x2+1x.
Asymptotes provide valuable information about the behavior of functions and are essential for understanding the overall shape and characteristics of graphs.
...
Conclusion 📄
To sum up, the Applied Algebra we studied today will enable students to manipulate different algebraic functions to understand real-world situations. We’ve outlined the curriculum for this article in detail, including the main subjects, which are the functions, graphs, and the mathematical properties. We have also outlined a class survey model that will aid students in preparation for the coming subjective assessment within a period of two weeks, recommending other resources and tips for reliability. By properly understanding what is covered in this module and faithfully following the study guide, students can steer the assessment confidently, and at the same time, use their learned knowledge to solve such issues across different disciplines.